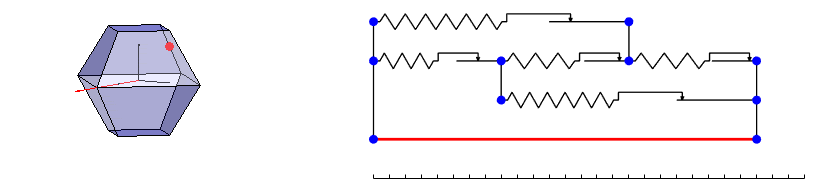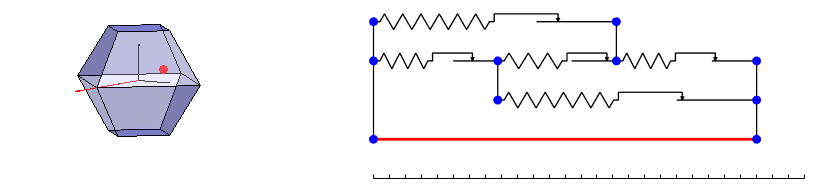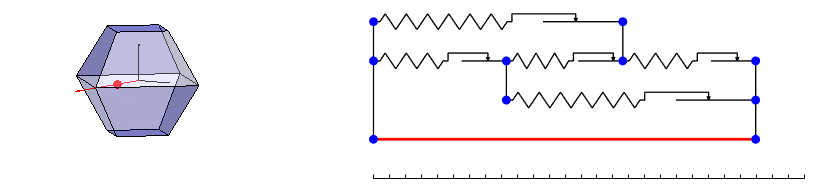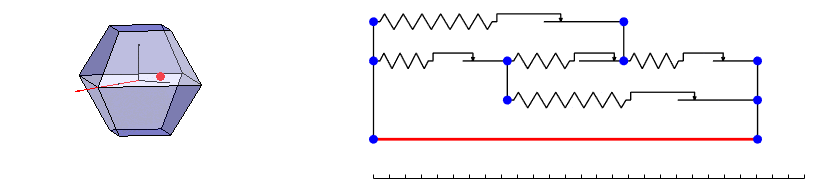
Moreau's Sweeping Process
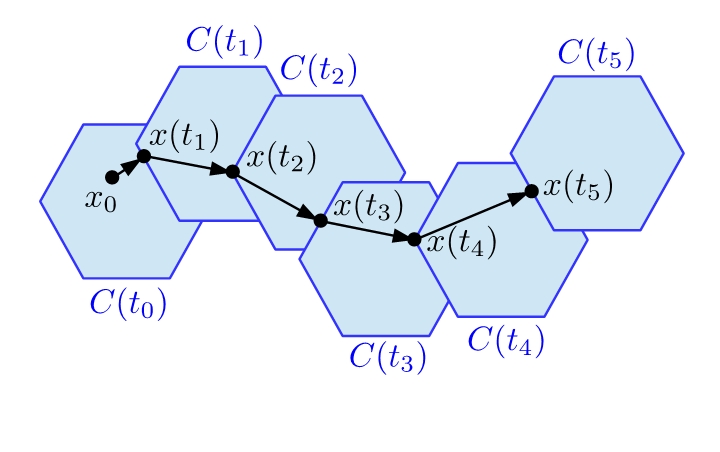 Catch-up algorithm (consecutive projections) is used to find a discrete approxtimation to a solution of a sweeping process.
Catch-up algorithm (consecutive projections) is used to find a discrete approxtimation to a solution of a sweeping process.
In late 1960’s French mathematician J.-J. Moreau introduced a method of solving nonsmooth evolution problems with applications in elastoplasticity. He used differential inclusions of the type $$-x’ \in N_{C(t)}(x),$$ which he called the sweeping process. Following his method, I am studying properties of solutions, which appear in such processes. I also work on the application of sweeping process to networks of elastoplastic springs. For example, the following animation shows a network of 5 springs (right side) and the corresponding sweeping process (left side).